
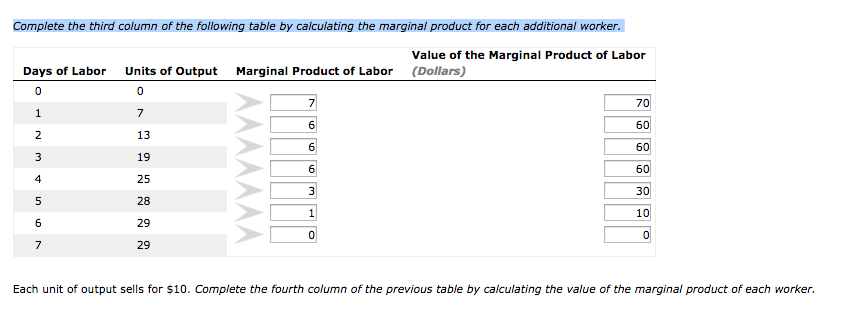
THE LAW OF VARIABLE PROPORTIONS AND THE LAW OF DIMINISHING PRODUCTĬonsider, for the sake of simplicity, a "canonical" short-run production function: y = f(K0, I) = F(I), where I (as before) is labor, y is the output of the firm, and K0 is a fixed given amount of another input that is conventionally defined as "capital" (or better services of a "capital good"). MPL X MR is called the marginal revenue product, and in this case its schedule (below the maximum of VAPL) represents the short-run firm’s demand curve for labor (a deeper analysis of these matters may be found in any standard microeconomic text).

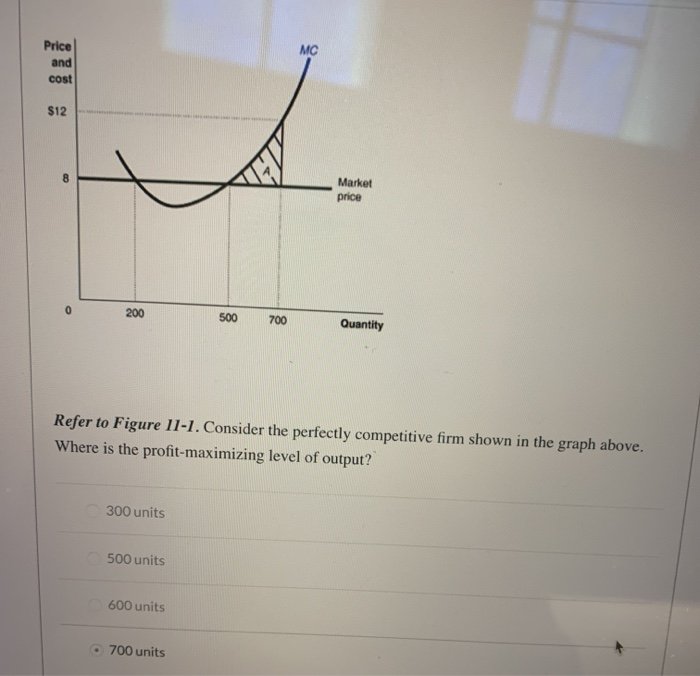
If the firm operates in a noncompetitive market for its product, profit maximization requires that MPL X MR = w, where MR is the marginal revenue generated by an extra unit of output (in perfect competition MR = p). The short-run firm’s demand curve for labor. With respect to labor (p and w are, respectively, the unit prices of output and labor), profit maximization requires that, at equilibrium, the real wage should be This is known as the principle of variation.
A direct implication is that as the relative prices of factors change, the proportion in which they are employed will change as well, in order to reestablish equality between marginal product and input prices. The concept of MPL has a central place in marginal productivity theory, since at the very core of this approach is the understanding that in a competitive market the remuneration of each productive factor should be equal to its marginal contribution to production. THE MARGINAL PRODUCT OF LABOR AND THE DISTRIBUTION OF INCOME Although the same name is generally given to the two definitions reported above-and what follows will con form to this general use-it would be more appropriate to call the former (the discrete case) the "marginal product of labor," while the latter (the continuous case) would be more appropriately defined as the "marginal productivity of labor." These definitions may be easily extended to the case of joint production, that is, when a firm, for a given combination of inputs, produces more than one output (the so-called multiproduct firm).


 0 kommentar(er)
0 kommentar(er)
